Choose Area of Interest
SEARCH SOFTWARE
AVS ENGINEERS and ISID STRUCTURES’ Structural Design of the Fairmont Palace Udaipur Luxury Hillside Resort Organization: AVS ENGINEERS and ISID STRUCTURES Project Name: Structural Design of Fairmont Palace Udaipur Luxury Resort Location: Udaipur, Rajasthan, India Project Phase: Completed July 2025...
by Ashley Fee
Egis’ Digital Collaboration for the Canal Seine Nord Europe Project Organization: Egis Project Name: Canal Seine Nord Europe – Digital Collaboration and Sustainability Initiative Location: Hauts-de-France region, France Project Phase: Ongoing infrastructure development with digital collaboration Expected Completion Date: Long-term...
by Paul Rotter
APD Global Engineering’s Digital Substation Design for Perth’s Power Upgrade Organization: APD Global Engineering Project Name: PTA Sutherland St. Load Upgrade – Digital Substation Design and Infrastructure Modernization Location: Perth, Western Australia Project Phase: Ongoing construction with digital engineering implementation...
by Eleanore Nguyen-Locke
Hyundai Engineering’s STAAD-Driven Automation for Modular and Precast Structures Organization: Hyundai Engineering Project Name: Design Automation System for Modular Transport and Precast Concrete Structures Location: Seoul, South Korea Project Phase: Ongoing digital transformation Expected Completion Date: Currently in active deployment...
by Ashley Fee
Italferr’s AI-Powered Digital Twin for Serravalle Tunnel Organization: Italferr S.p.A. Project Name: Digital Twin Inspection and Maintenance System for the Serravalle Tunnel Location: Serravalle Tunnel, part of the Terzo Valico dei Giovi railway between Genoa and Milan, Italy Project Phase:...
by Oana Crisan
Italferr’s Palermo–Catania–Messina Railway Transformation Organization: Italferr (in collaboration with national and regional rail stakeholders) Project Name: New Palermo–Catania–Messina Railway Connection Location: Sicily, Italy Project Phase: Ongoing infrastructure modernization Expected Completion Date: Currently in progress as part of large-scale national infrastructure...
by Paul Rotter
Caiziba Pumped-Storage Power Station Organization: Shanghai Investigation, Design & Research Institute Co., Ltd. (SIDRI) Project Name: Caiziba Pumped-Storage Power Station Location: Fengjie County, Chongqing, China Project Phase: Construction and Commissioning Expected Completion Date: Not specified Estimated Project Cost: Not specified...
by Gavin England
Sarawak Sabah Link Road Phase 2 Organization: Jabatan Kerja Raya Sarawak (JKRS) Project Name: Sarawak Sabah Link Road Phase 2 Location: Limbang and Lawas, Sarawak, Sarawak Project Phase: Construction Expected Completion Date: June 23, 2029 Estimated Project Cost: MYR 7.15...
by Megan Tigue
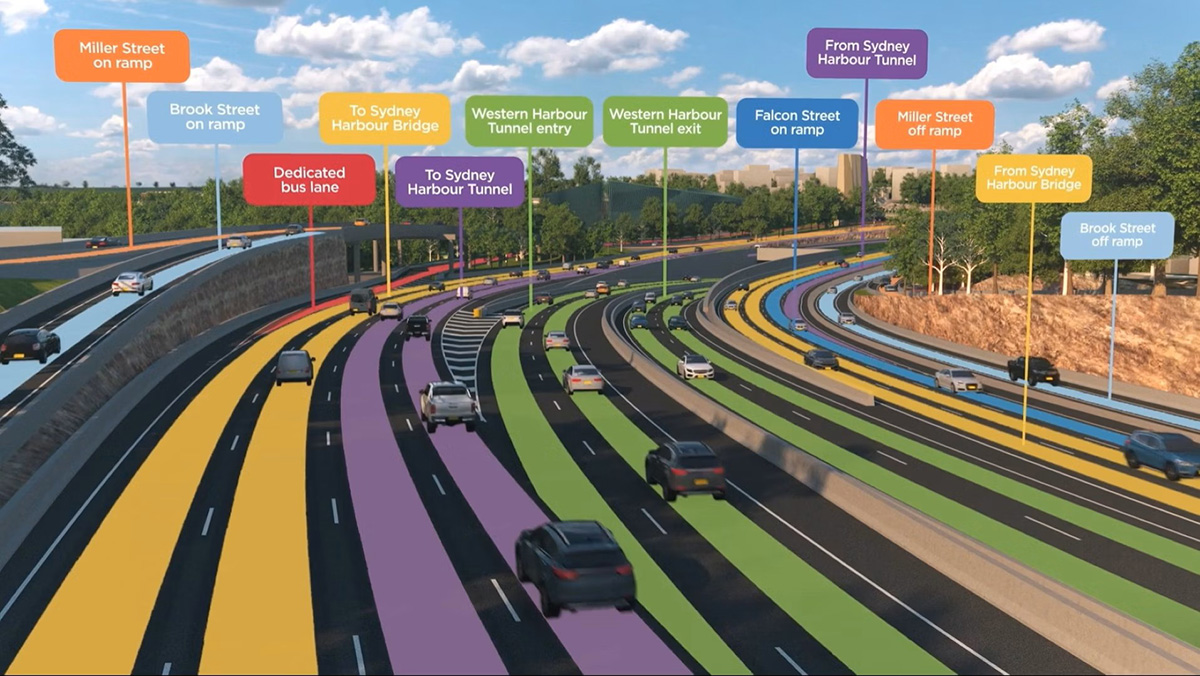
Warringah Freeway Upgrade Project Organization: Arcadis Project Name: Warringah Freeway upgrade Location: Sydney, New South Wales, Australia Project Phase: Construction Expected Completion Date: December 31, 2026 Estimated Project Cost: AUD 1.18 billion Bentley Software: Bentley LumenRT, iTwin Capture, iTwin Experience,...
by Megan Tigue