A shell structure is thin in one direction and long in the other two directions. Shell structures are used in a variety of architectural and industrial applications as they are lightweight, can span large areas, and can be designed to be strong and stiff. Examples of shell structures include stadium roofs, airplane fuselages, ship hulls, automobile hoods and body panels, bridge decks, and oil tanks.
Shell structures are highly dependent on the geometry and boundary conditions. Any small change can have a dramatic effect. When carrying load, the shell structure may be in membrane, bending or mixed strain states, and internal and boundary concentrated strain layers may be present. Curvature is important for the load carrying characteristics of shell structures. A flat shell structure (a plate) subjected to pressure is flexible because it resists the loading only in bending action. A curved shell structure can resist loading in bending and membrane actions and then is much stiffer. Before a building or container that employs a shell structure can be constructed, engineers must analyze the shell structure to ensure it will withstand the necessary loads.
Low-order 3-node and 4-node Mixed Interpolation of Tensorial Components (MITC) shell finite elements have been used abundantly in engineering practice for many years very successfully to analyze shell structures. MITC shell elements interpolate the transverse shear strains separately from the displacements to alleviate transverse shear locking [1,2]. They perform well in all cases except for bending dominated thin shell structures meshed with elements that are highly distorted out-of-the-plane (highly warped elements). In this case, MITC shell elements do not behave optimally in membrane action, so that a fine mesh of MITC shell elements may need to be used for accurate results.
In ADINA 9.5.1, a new MITC+ formulation is available for 3-node and 4-node shell elements, referred to, respectively, as MITC3+ and MITC4+ shell elements, as shown in Figure 1.
Figure 1 3-node and 4-node MITC+ (MITC3/4+) shell elements
The new MITC+ shell element alleviates shear locking using the same assumed transverse shear strain field as the original MITC shell element and displays better membrane behavior using a new assumed membrane strain field. As a result, the new MITC+ shell element passes all fundamental tests, even for meshes with highly distorted elements and shows practically optimal convergence behavior [3 – 5].
Real engineering structures resist loads in mixed membrane/bending action. However, in this Tech Brief, we focus on tough shell benchmark problems, in which the shell structure resists loads in only membrane action or only bending action, in order to study the behavior of the shell elements in these separate actions. The behavior in combined membrane and bending actions will then be a superposition of the individual behaviors.
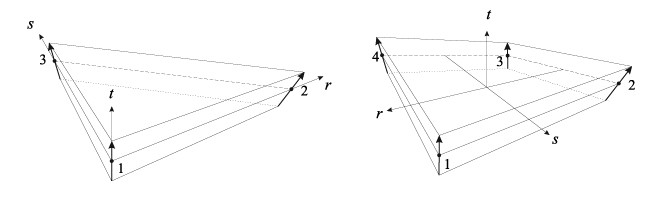
Figure 2 shows a tough behavior-encompassing shell benchmark problem of a doubly-curved cooling tower (the “hyperbolic shell” problem). If the top and bottom ends of the cooling tower are fixed, the problem is membrane dominated. But if the ends of the cooling tower are free, the problem is bending dominated.
Figure 2 Cooling tower problem
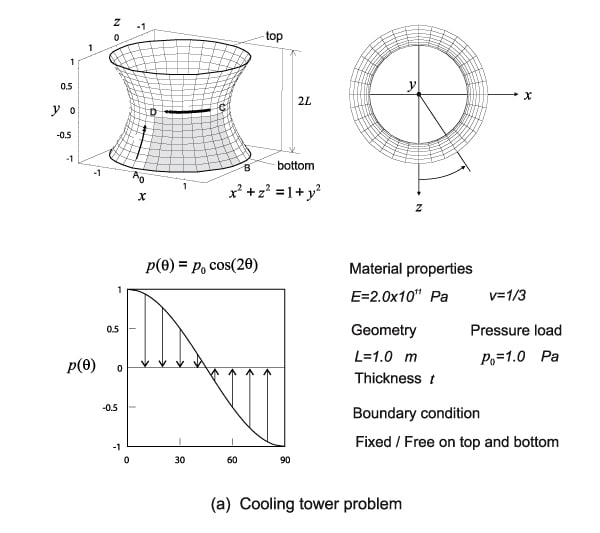
To assess the performance of shell elements, an appropriate error measure must be used. For mixed formulations, the s-norm is used [6]. The error measure, 
, is given by:
where 
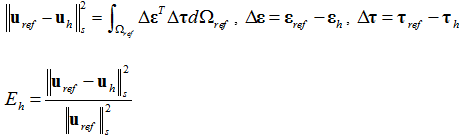
is the displacement, 
is the strain, and 
is the stress. We integrate over the 3D shell domain.
The s-norm measure used here is the difference between the exact and approximate strains (and the exact and approximate stresses). It can be used for membrane dominated, bending dominated and mixed problems.
The cooling tower in Figure 2 is meshed with 4-node shell elements. Figure 2b shows the undistorted and distorted meshes used. For the distorted meshes, the sides are subdivided with ratio 1 : 2 : … : N, where N is the number of subdivisions.
Figure 3 shows the convergence curves for the fixed boundary (membrane dominated) case. Both MITC4 and MITC4+ shell elements perform well for undistorted and distorted meshes.
Figure 3 Convergence curves of the cooling tower problem with fixed boundaries (membrane dominated). The s-norm is used.
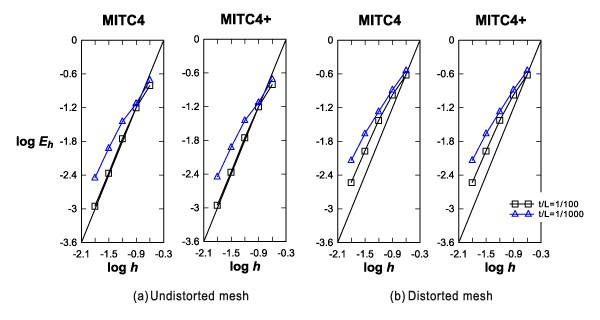
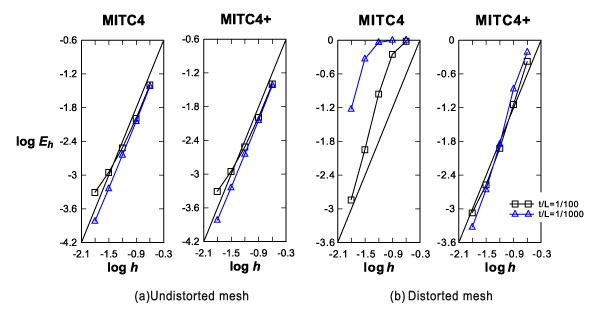
Figure 4 shows the convergence curves for the free boundary (bending dominated) case. For undistorted meshes, both MITC4 and MITC4+ shell elements perform well. However, for distorted meshes, a fine mesh of MITC4 shell elements must be used for accurate results, whereas MITC4+ shell elements give practically optimal convergence behavior.
Figure 4 Convergence curves of the cooling tower problem with free boundaries (bending dominated). The s-norm is used.
Because the new MITC+ shell elements are continuum mechanics-based elements with a strong theoretical basis, they can be directly extended to nonlinear analysis in a manner that is consistent with the principle of virtual work.
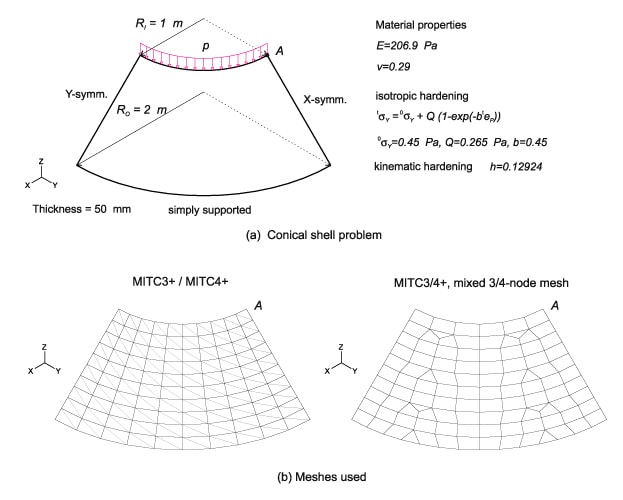
Figure 5 shows a large strain analysis of a conical shell structure that buckles with material and geometric nonlinearities. The conical shell structure is meshed with 3-node triangular MITC3+ shell elements, 4-node quadrilateral MITC4+ shell elements, and a mixture of 3-node and 4-node triangular and quadrilateral MITC3/4+ shell elements. Figure 5b shows the meshes used.
Figure 5 Large strain analysis of a conical shell
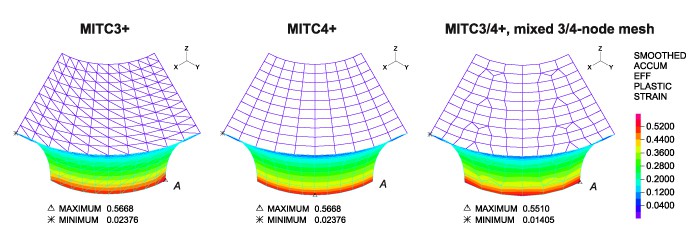
The movie above, and Figures 6 and 7 show the results. As can be seen, good results are obtained for all meshes of the new MITC+ shell elements.
Figure 6 Load-displacement curve of the conical shell
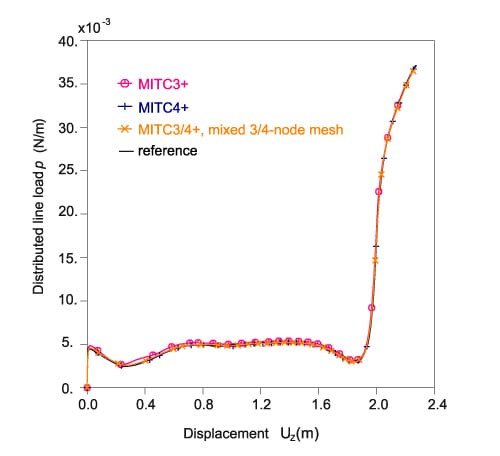
Figure 7 Accumulated plastic strain at final state of the conical shell
In this Tech Brief, we show that the new MITC+ shell elements in ADINA give practically optimal convergence behavior, even when the shell elements are highly distorted.
The new MITC+ shell elements in ADINA are very powerful and strengthens the ADINA offering for accurate, reliable and robust analysis of shell structures.
Keywords:
Shell elements, element distortions, distortion sensitivity, large displacements, large strains, MITC, MITC+, buckling, membrane dominated, bending dominated, mixed strain state, mixed formulation, convergence curves, practically optimal
