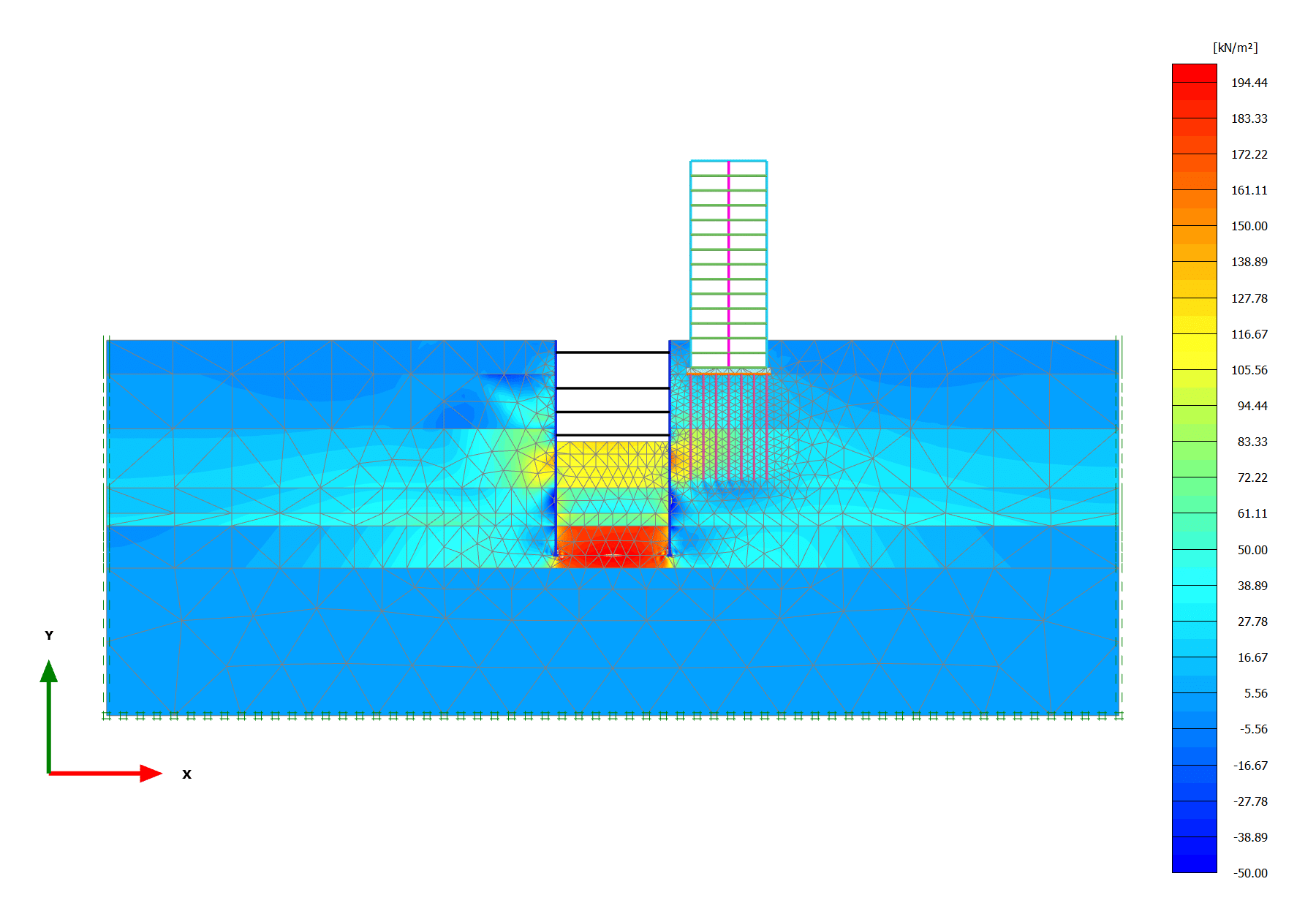
K0 Procedure initial calculation type
Gravity Loading initial calculation type
Field Stress initial calculation type
Flow Only initial calculation type
Plastic deformation calculation type
Safety deformation calculation type
Consolidation deformation calculation type
Dynamic deformation calculation type
Fully coupled flow-deformation calculation type
Phreatic Level pore pressure calculation type
Use Pore Pressures from Previous Phase pore pressure calculation type
Steady-State Groundwater Flow pore pressure calculation type
Transient Groundwater Flow pore pressure calculation type
Ignore Temperature thermal pressure calculation type
Earth Gradient thermal pressure calculation type
Steady-State Thermal Flow thermal pressure calculation type
Use temperatures from Previous Phase thermal pressure calculation type
Transient Thermal Flow thermal pressure calculation type